Remember that one late summer afternoon when you were buried in your math textbook, struggling to comprehend the concept of calculating the area of composite figures? The textbook illustrations seemed to dance before your eyes, and the formulas felt like a foreign language. You yearned for a guiding hand, a key to unlock the secrets of these complex shapes. Well, today, we’re going to delve into the world of composite figures and empower you with the tools to conquer any area calculation challenge. Forget about the frustration; we’ll equip you with the knowledge and confidence to ace your next homework assignment.
Image: www.teacherspayteachers.com
This article will serve as your comprehensive guide to understanding and tackling problems related to the area of composite figures. We’ll explore the fundamentals, break down complex concepts into easily digestible steps, and equip you with the practical skills needed to excel in your math class. Get ready to embrace the challenge and unlock the secrets of composite figures.
Understanding Composite Figures: A Building Block Approach
Imagine a puzzle, but instead of colorful pieces, you’re working with geometric shapes. Composite figures are just that — shapes formed by combining two or more basic geometric figures like rectangles, triangles, circles, and squares. Think of a house: its roof might be a triangle, its walls rectangular, and its windows circular. When we calculate the area of a composite figure, we’re essentially finding the total space enclosed by all the individual shapes that make it up.
Mastering the art of calculating the area of composite figures is like having a secret weapon in your math arsenal. It’s about breaking down the complex into simpler components, utilizing familiar formulas to find the area of each individual shape, and then diligently adding them together to obtain the total area. You’ll see that it’s not an intimidating process; it’s a systematic and rewarding approach to solving intricate problems.
Diving Deeper into Area Calculations
Deconstructing the Composite
The first principle to grasp is that every composite figure can be dissected into its fundamental components. Imagine it like separating a jigsaw puzzle into its individual pieces. Once you can identify the basic shapes that make up the composite figure, you can focus on calculating their individual areas.
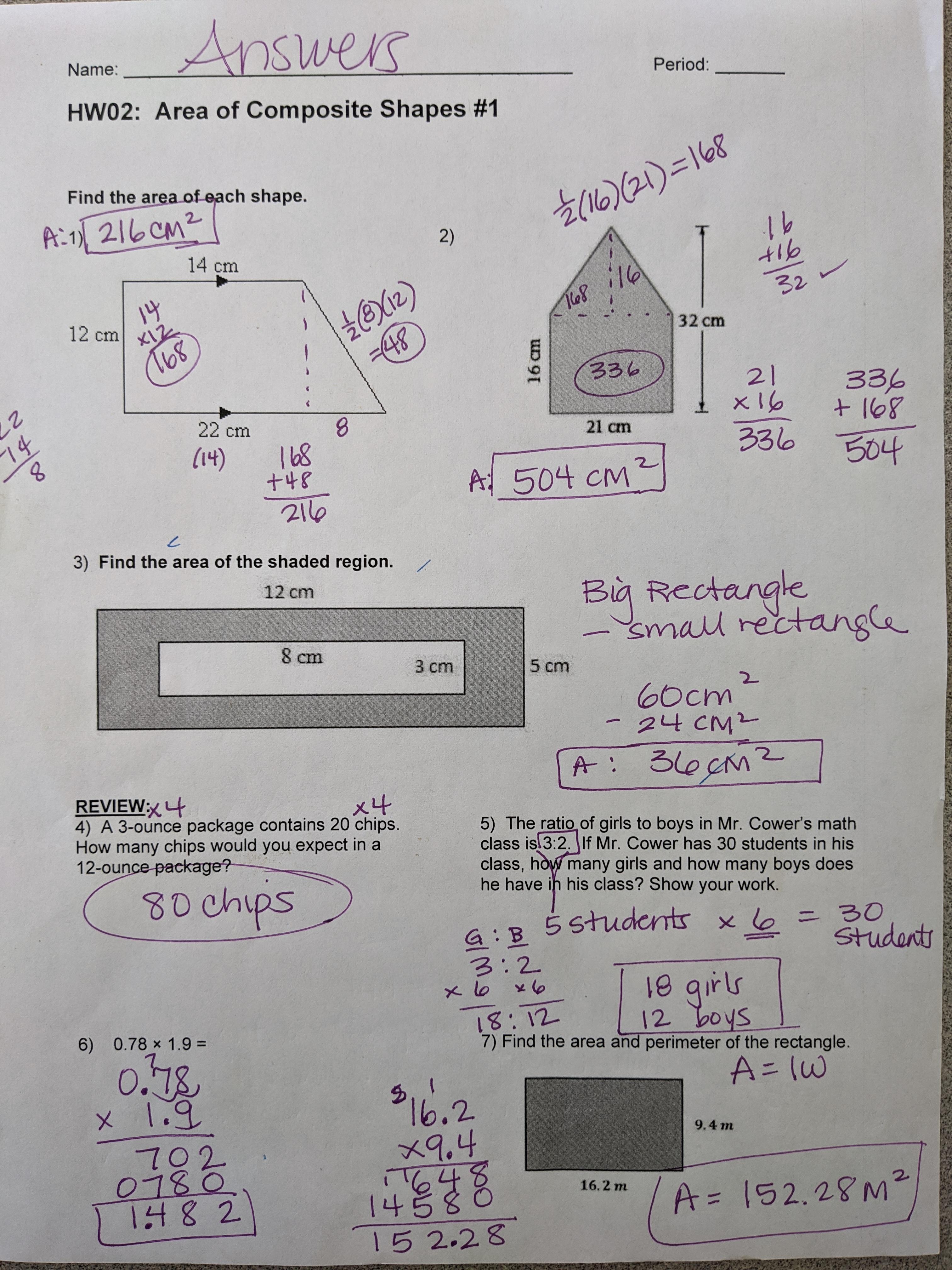
Image: du31732101.blogspot.com
Harnessing Familiar Formulas
Remember those tried-and-true formulas for calculating the areas of simple shapes like squares, rectangles, triangles, and circles? They are the cornerstones of calculating the area of composite figures. For squares and rectangles, you’ll need the formulas Area = length x width and Area = side x side. For triangles, it’s Area = 1/2 x base x height. And for circles, Area = π x radius². These formulas will become your trusted tools as you break down composite figures.
Strategic Decomposition and Aggregation
Once you’ve determined the individual shapes within the composite figure, carefully calculate the area of each shape using the appropriate formula. Then, comes the final step: summing up the areas of all the individual shapes to obtain the total area of the composite figure. It’s like adding the pieces of a jigsaw puzzle back together, but in this case, you’re adding up areas to find the whole.
Essential Tips and Techniques
The beauty of calculating the area of composite figures lies in its adaptability. Depending on the shape you are presented with, there might be multiple approaches you can take to break it down.
1. Divide and Conquer:
When faced with a seemingly complex composite figure, think about how you can divide it into simpler, more manageable shapes. A well-placed line could transform a complicated shape into a rectangle and a triangle, making calculations much easier.
2. Pay Attention to Overlaps:
Sometimes, composite figures might have overlapping regions. It’s important to be mindful of these overlaps to avoid counting the area of these sections twice. Carefully analyze the diagram and make sure you’re only calculating each area once.
3. Combine Shapes:
In some cases, you might be able to combine two or more shapes to form a new, simpler shape before calculating the area. This can streamline calculations and prevent unnecessary steps.
Frequently Asked Questions
Q1. Can you give me an example of a composite figure problem?
Imagine a rectangular garden with a semicircular flower bed in one corner. To find the area of the garden, excluding the flower bed, you’d need to calculate the area of the rectangle and subtract the area of the semicircle.
Q2: How do I identify the individual shapes within a composite figure?
Look for familiar geometric shapes like rectangles, triangles, circles, or squares. Focus on the lines, curves, and angles that define these shapes within the composite figure.
Q3: What if I’m dealing with an irregular composite figure?
Even for irregular composite figures, you can break them down into simpler shapes. You might need to get creative with your lines of division but the principle remains the same.
Homework 3 Area Of Composite Figures Answer Key
Unlocking the Power of Composite Figure Calculations
Mastering the concept of area and composite figures empowers you with a new level of understanding in geometry and problem-solving. It’s not about memorizing formulas but about developing a strategic approach to dissect and analyze complex shapes. You’ve dived into the world of composite figures, learning how to break them down, apply basic formulas, and calculate their total area.
Now, are you ready to tackle your next homework assignment with confidence? Share your thoughts and let me know if you’re eager to dive deeper into the world of composite figures.





